`color{red}{"If elements of a row (or column) are multiplied with cofactors of any
other row (or column), then their sum is zero."}`
For example,
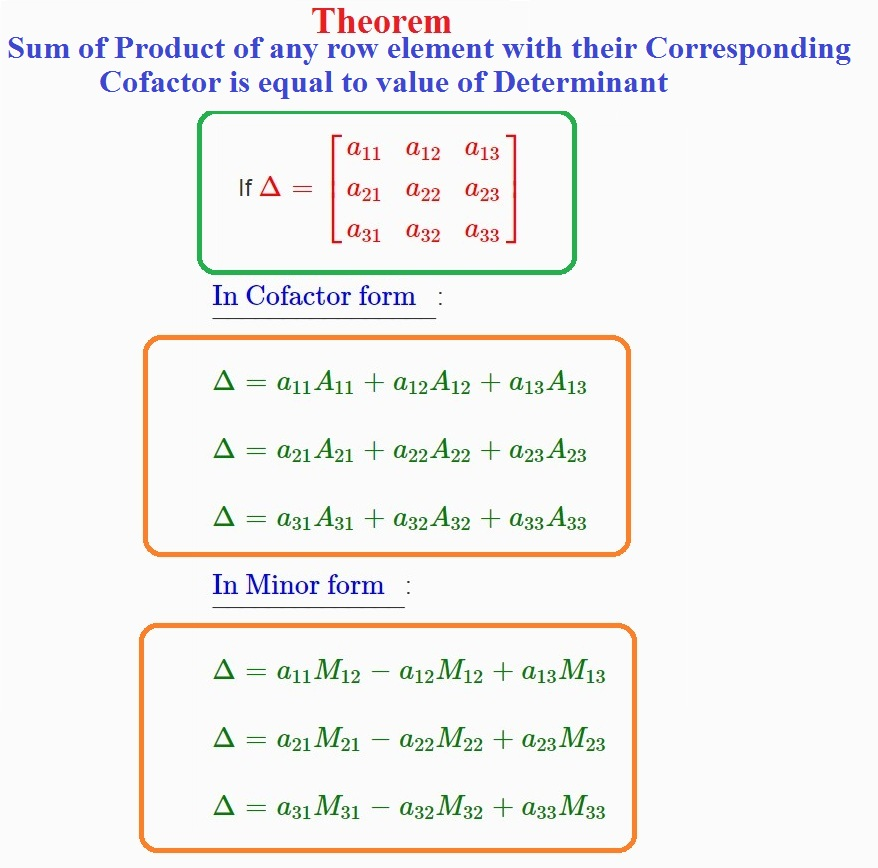
Let `color {red} {Delta = [ (a_11 ,a_12 ,a _13 ),( a_21 , a_22 , a_23 ), ( a_31 ,a _32 , a_33 )]}`
` Δ = a_11 A_21 + a_12 A_22 + a_13 A_23`
`= a_11 (-1)^(1+1) | ( a_12, a_13), ( a_32, a_33) | + a_12 (-1)^(1+2) | (a_11 , a_13), ( a_31 , a_33) | + a_13 (-1)^(1+3) | (a_11, a_12), ( a_31, a_32) |`
`= | (a_11, a_12, a_13 ), ( a_11 , a_12, a_13) ,( a_31 ,a_32 , a_33) | = 0` (since `R_1` and `R_2` are identical)
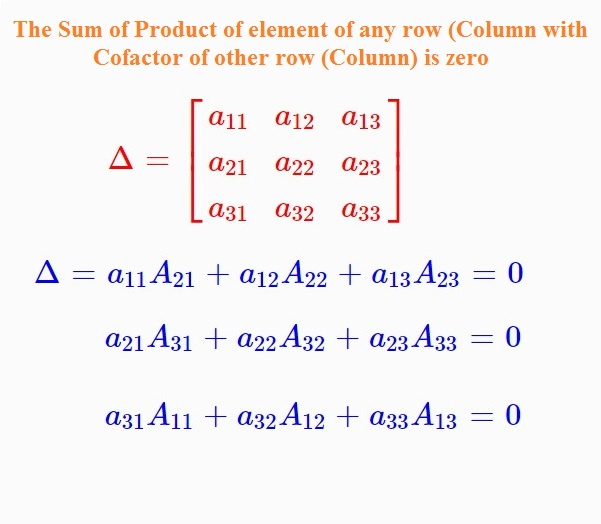
The Sum of Product of element of any row (column) with Cofactor of other row (Column) is zero.
`color{blue} { a_11A_21 +a_12 A_22 + a_13 A_23 = 0}`
`color {blue} {a_21A_31 +a_22A_32 + a_23 A_33 = 0}`
`color {blue} {a_31A_11 + a_32 A_12 + a_33A_13 =0}`
Similarly, we can try for other rows and columns
`color{red}{"If elements of a row (or column) are multiplied with cofactors of any
other row (or column), then their sum is zero."}`
For example,
Let `color {red} {Delta = [ (a_11 ,a_12 ,a _13 ),( a_21 , a_22 , a_23 ), ( a_31 ,a _32 , a_33 )]}`
` Δ = a_11 A_21 + a_12 A_22 + a_13 A_23`
`= a_11 (-1)^(1+1) | ( a_12, a_13), ( a_32, a_33) | + a_12 (-1)^(1+2) | (a_11 , a_13), ( a_31 , a_33) | + a_13 (-1)^(1+3) | (a_11, a_12), ( a_31, a_32) |`
`= | (a_11, a_12, a_13 ), ( a_11 , a_12, a_13) ,( a_31 ,a_32 , a_33) | = 0` (since `R_1` and `R_2` are identical)
The Sum of Product of element of any row (column) with Cofactor of other row (Column) is zero.
`color{blue} { a_11A_21 +a_12 A_22 + a_13 A_23 = 0}`
`color {blue} {a_21A_31 +a_22A_32 + a_23 A_33 = 0}`
`color {blue} {a_31A_11 + a_32 A_12 + a_33A_13 =0}`
Similarly, we can try for other rows and columns
 Find the area of the triangle whose vertices are (3, 8), (– 4, 2) and (5, 1).
Find the area of the triangle whose vertices are (3, 8), (– 4, 2) and (5, 1).